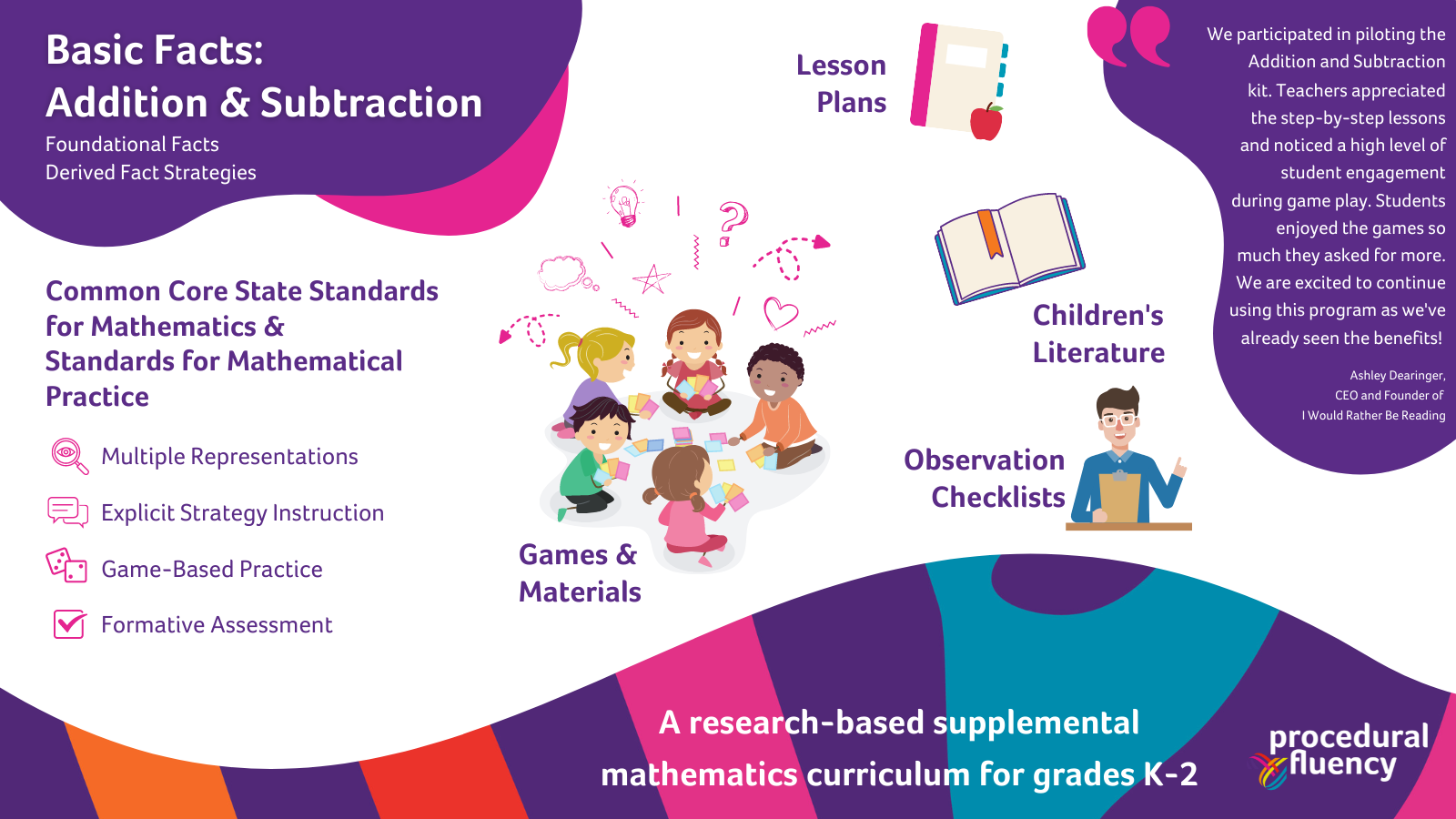
CTL’s Procedural Fluency Model is a Kindergarten through Grade 5 program that helps students develop conceptual understanding of operations and basic fact fluency. Using explicit strategy instruction, game-based practice and embedded formative assessment, students develop fluency with the basic facts. Each kit provides a teacher all the materials needed, including games, cards, dice, two-color counters, books for literacy connections, work mats, teacher guidebook, description cards, and Intervention/Out of School Time guide.
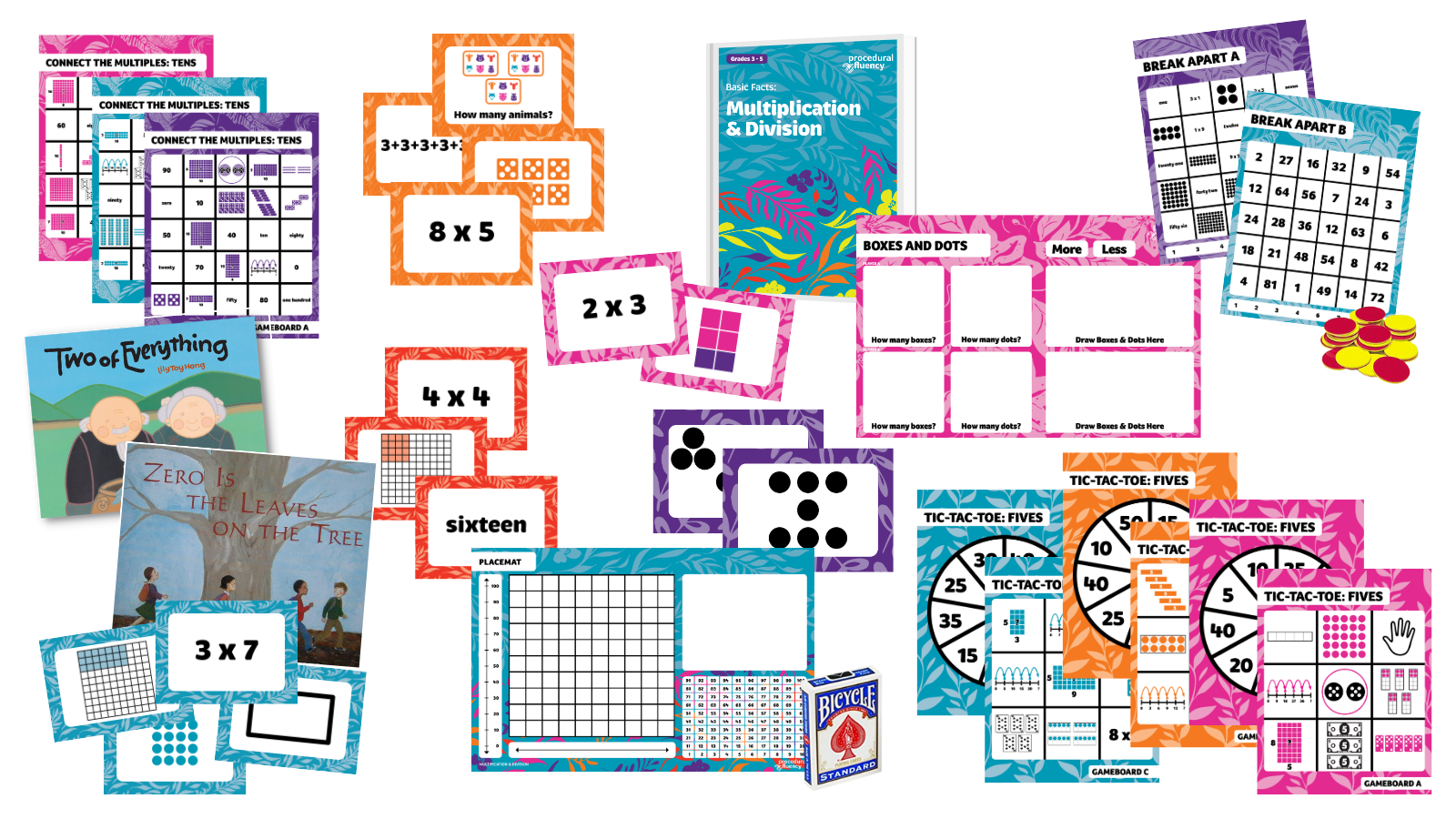
Kits are made out of tear resistant material and each module is designed to make it easy on the teacher to keep track of the different games in each module. Students can easily distinguish cards based on color and/or design. The addition/subtraction kit has an animal print theme and the multiplication/division kit has a flora and fauna theme. The cards and themes were designed to be accessible for students with color difficulties.
Basic Facts:
Addition & Subtraction
Basic Facts:
Multiplication & Division
Multiple Representations
The vibrant materials were designed so that students have the opportunity to see quantities represented in multiple ways:
- Dot Patterns
- Pictures
- Tens Frames
- Number Lines
- Standard Form and Word Form (each number 0 – 20)
During game play, students build connections between the representations in order to further develop their conceptual understanding and number sense.

Multiple Representations
The materials were designed so that students have the opportunity to see quantities represented in multiple ways:
- Dot Patterns
- Pictures
- Set and Area Arrays
- Number Lines
- Symbolic Expressions
- Standard Form and Word Form
During game play, students build connections between the representations in order to develop their conceptual understanding and number sense.
game-based practice
As a primary focus of this resource, game-based practice provides numerous opportunities for all students to:
- practice strategy application and
- develop a positive disposition towards mathematics.
Rather than expecting students to respond correctly on timed tests, teachers shift their focus toward students who are engaged in strategy discussion with their peers.
Games are designed to be easily adapted for use with all students.


formative assessment
As small groups of students are engaged in playing games, the teacher monitors:
- accuracy,
- efficiency, and
- appropriate and flexible use of strategies.
Using the provided Observation Checklists and question routines, teachers track individual student progress.
The game-based practice and formative assessment components emphasize the developmental and research-based approach of the program.

Professional Learning & coaching
All of CTL’s professional learning models meet the Learning Forward Professional Learning Standards for effective student learning. The most recent findings show a combination of curriculum and professional learning produce the greatest impact on student learning (Lynch, Gonzalez, & Pollard, 2018). Professional learning experiences focus on teacher understanding of the conceptual progression, analysis of instruction, and reflection. CTL offers a Foundational Training for all teachers.